Simpson’s Diversity Index is a way to measure the diversity of species in a community.
Denoted as D, this index is calculated as:
D = Σni(ni-1) / N(N-1)
where:
- ni: The number of organisms that belong to species i
- N: The total number of organisms
The value for Simpson’s Diversity Index ranges between 0 and 1. The higher the value, the lower the diversity.
Since this interpretation is a bit counterintuitive, we often calculate Simpson’s Index of Diversity (sometimes called a Dominance Index), which is calculated as 1 – D. The higher the value for this index, the higher the diversity of species.
We can also calculate Simpson’s Reciprocal Index, which is calculated as 1/D. The lowest value for this index is 1 and the highest value is equal to the number of species.
For example, if there are 7 different species then the max value for this index would be 7. The higher the value for this index, the greater the diversity of the species.
The following step-by-step example shows how to calculate these various indices for a given community.
Step 1: Collect the Data
Suppose a biologist wants to measure the diversity of species in a local forest. She collects the following data:

Step 2: Calculate N
Next, she can calculate the total number of organisms.
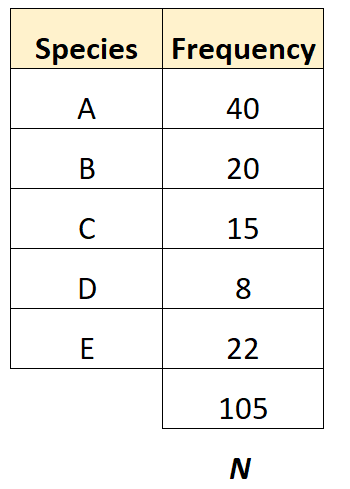
There are 105 total organims.
Step 3: Calculate ni(ni-1)
Next, she can calculate ni(ni-1). For example, the first species would be calculated as 40*(40-1) = 1,560. She can repeat this calculation for each species:

Step 4: Calculate Simpson’s Diversity Index
Lastly, we can use the following formula to calculate Simpson’s Index:
D = Σni(ni-1) / N(N-1)
Using the values we found earlier, Simpson’s Index can be calculated as:
D = 2,668 / (105*(105-1)) = 0.244.
We can also calculate Simpson’s Index of Diversity as 1 – D = 1 – 0.244 = 0.756.
We can also calculate Simpson’s Reciprocal Index as 1 / D = 1 / .244 = 4.09.
Additional Resources
Feel free to use this Simpson’s Diversity Index Calculator to automatically calculate Simpson’s Diversity Index for any dataset.
